We explain what polygons are and how these geometric figures are classified. Also, what are its characteristics and examples.
What are polygons?
A polygon is any flat geometric figure that is composed of three or more straight lines that make up a closed figure . These consecutive lines are called sides and a polygon is also characterized by having three or more angles and vertices and being crossed by diagonals (except for the triangle).
Although many geometric figures are polygons, not all are. Some examples of polygons are triangles, squares, rhombuses, rectangles , hexagons, pentagons, among many others.
The word polygon comes from the Greek: polygons and derives from the word poly , which means many , and from gonos, which means sides. Each polygon is usually named according to the number of sides it has.
Characteristics of polygons
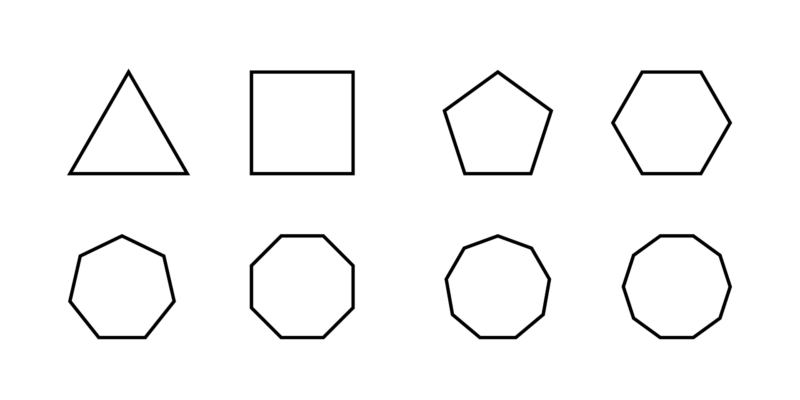
The main characteristics of polygons are:
- They are two-dimensional figures . Every polygon has two dimensions: height and width. Two-dimensional figures do not have the third dimension, which is depth, therefore polygons have neither volume nor weight.
- They are closed figures . Every polygon, to be considered as such, must be closed, that is, it must have angles and the figure must not be open. If in a figure one of the sides does not join at its end with another, it is not a polygon.
- They are made up of segments or straight lines . The sides or lines of the polygon must be, without exception, straight. If any of the parts or sides of a geometric figure is curved, it is no longer a polygon.
Elements of a polygon
All polygons are characterized by having:
- Sides . They are the lines that make up the figure. A polygon must have at least three sides and there is no maximum number of sides. A polygon can be, for example, a triangle (polygon with three sides), a pentagon (polygon with five sides), an octagon (polygon with eight sides), among many others.
- Vertices . They are the unions or intersections that occur when joining two sides or lines of the polygon. The number of vertices of a polygon is equal to the number of sides of the figure.
- Angles . They are the angles that are formed between two lines or sides of the polygon and that have a certain inclination or graduation.
- Exterior angles . They are the angles that are formed between one of the sides and a line outside the polygon.
- Diagonals . They are lines that can be joined within the polygon between a vertex and another non-consecutive. The number of diagonals that can be drawn in a polygon will depend on the number of sides. The more sides a polygon has, the more diagonals can be drawn. The triangle is the only polygon that has no diagonals.
- Center . It is the point equidistant from all sides and vertices of a regular polygon.
- Apothem . It is the distance between the center of the polygon and any of the sides.
- center angle . It is the angle that its vertex has at the center of the polygon and its sides are formed by joining that center with two consecutive vertices of the polygon.
Polygon Types
 Polygons can be classified according to certain criteria.
Polygons can be classified according to certain criteria.According to its shape :
- simple polygon . It does not allow its edges to cross, that is, it forms a figure in which none of the sides intersect. Can be:
- convex polygon . Polygon in which at least one of its interior angles measures less than 180º.
- concave polygon . Polygon in which at least one of its internal angles measures more than 180º.
- complex polygon . Their edges (or sides) intersect. It is easily recognizable because one of the lines crosses over another line of the polygon.
- Regular polygon . It has all the sides and interior angles of the same length.
- Irregular polygon . It has sides and interior angles of different lengths.
According to the number of sides :
Some examples are:
- Triangle (polygon with three sides).
- Quadrilateral (polygon with four sides).
- Pentagon (five-sided polygon).
- Hexagon (polygon with six sides).
- Heptagon (polygon with seven sides).
- Octagon (polygon with eight sides).
- Nonagon (polygon with nine sides).
- Decagon (polygon with ten sides).
- Pentadecagon (polygon with fifteen sides).
- Isodecagon (polygon with twenty sides).
- Triacontagon (thirty-sided polygon).
- Pentacontagon (fifty-sided polygon).
- Hectagon (polygon with one hundred sides).
How do you find the perimeter and area of a polygon?
The perimeter of a polygon is the contour of the figure and is obtained by adding all its sides . If it is a regular polygon (whose sides are of the same measure), a multiplication can be performed. In the case of irregular polygons, it is not possible to perform a multiplication because their sides do not have the same length and the addition must be done directly.
To obtain the area of a polygon, the type of polygon with which you are working must be taken into account, since the area is calculated differently according to each type of geometric figure . The formula will change depending on whether it is a triangle, a square, or any other regular or irregular polygon.
For example:
- Area of a rectangle = bxh (base times height).
- Area of a square = lxl (side by side).
- Area of a triangle = (bxh) 2 (base times height divided by two).
- Area of a rhombus = (D xd) 2 (major diagonal by minor diagonal divided by two).
Polygons in everyday life
 Polygons are geometric shapes that are both in nature and creations of the human being . Some examples are:
Polygons are geometric shapes that are both in nature and creations of the human being . Some examples are:
- In the starfish .
- In flowers and fruits.
- In the honeycombs.
- In the snow crystals.
- On traffic signs.
- In buildings such as houses and buildings.
- In objects such as books, pillows, soccer balls, and boxes.
- At the pyramids.
- In furniture such as tables, benches and bookcases.
- On roads and paths.
- In clothing such as scarves and t-shirts.
- On windows and doors.
- On the flags.
- In foods such as cakes, sandwiches, cheeses, and cookies.
- In tools such as hammers and nuts.
- In appliances such as refrigerators and microwaves.
- On computers, cell phones and tablets.
- In sweets and chocolates.
- In sheets, curtains and towels.
- In swimming pools and flowerbeds.
The above content published at Collaborative Research Group is for informational and educational purposes only and has been developed by referring reliable sources and recommendations from experts. We do not have any contact with official entities nor do we intend to replace the information that they emit.
Veronica is a culture reporter at Collaborative Research Group, where she writes about food, fitness, weird stuff on the internet, and, well, just about anything else. She has also covered technology news and has a penchant for smartphone stories. .
Leave a reply
Your email address will not be published. Required fields are marked *Recent post

Sport: What Is It, Types, Risks, Features, Characteristics and Examples

Dogs: Emergence, Features, Characteristics, Feeding and Breeds

Story: Definition, Elements, Structure, Features and Characteristics

